3D Coordinate Geometry - Equation of a Plane

A plane is a flat, two-dimensional surface that extends infinitely far. A plane is the two-dimensional analog of a point (zero dimensions), a line (one dimension), and three-dimensional space. A plane in three-dimensional space has the equation

$$ax + by + cz + d=0,$$

where at least one of the numbers $a, b,$ and $c$ must be non-zero. A plane in 3D coordinate space is determined by a point and a vector that is perpendicular to the plane.

This wiki page is dedicated to finding the equation of a plane from different given perspectives.

A plane in 3D coordinate space is determined by a point and a vector that is perpendicular to the plane. Let $P_{0}=(x_{0}, y_{0}, z_{0} )$ be the point given, and $\overrightarrow{n}$ the orthogonal vector. Also, let $P=(x,y,z)$ be any point in the plane, and $r$ and $r_{0}$ the position vectors of points $P$ and $P_{0},$ respectively. Now, if we let $\overrightarrow{n}=(a,b,c) ,$ then since $\overrightarrow{P_{0}P}$ is perpendicular to $\overrightarrow{n},$ we have

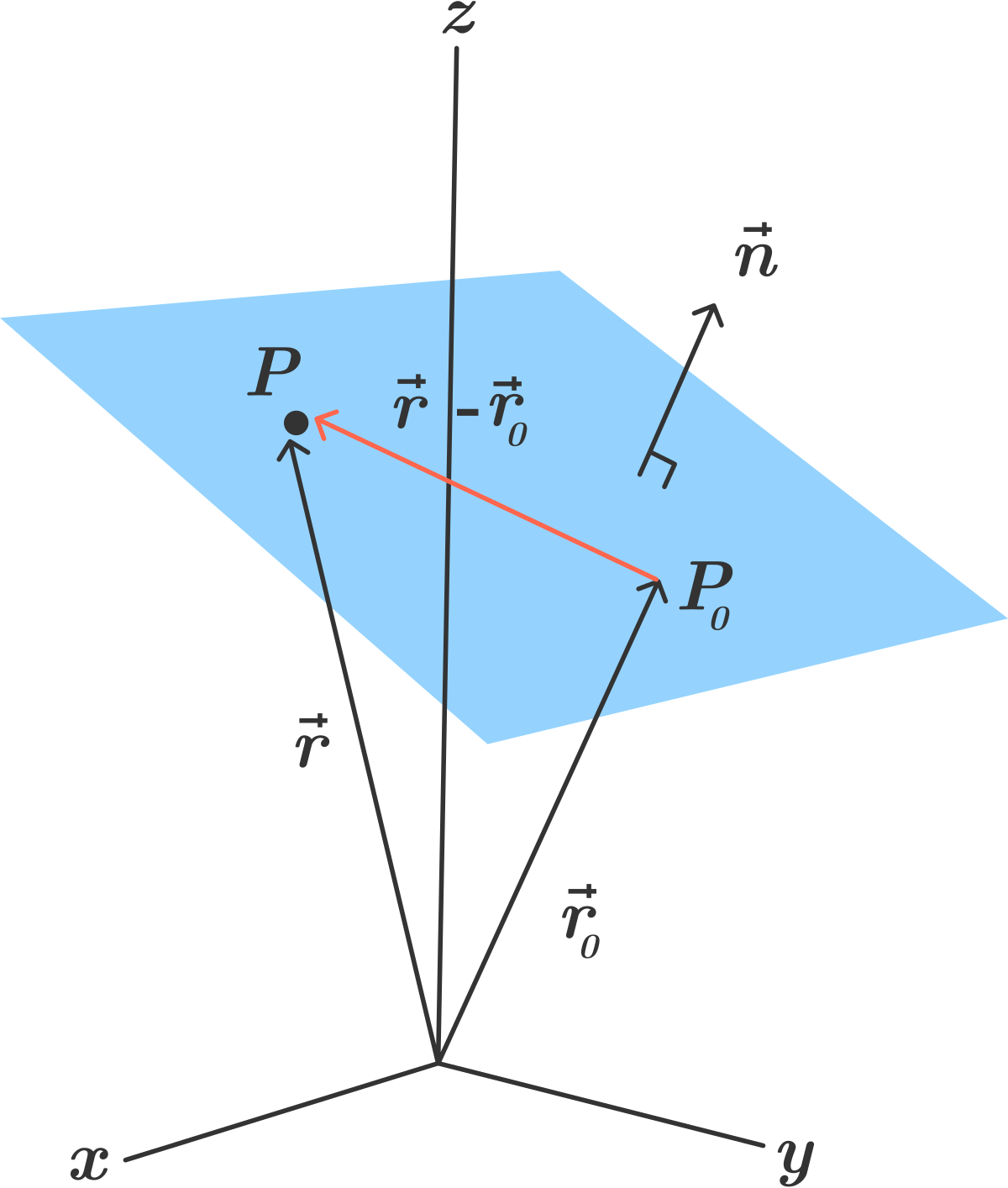
$$\begin{aligned} \overrightarrow{P_{0}P} \cdot \overrightarrow{n} &= (\overrightarrow{r}-\overrightarrow{r_{0}}) \cdot \overrightarrow{n} \\ &= (x-x_{0}, y-y_{0}, z-z_{0}) \cdot (a, b, c) \\ &= a(x-x_{0}) + b(y-y_{0}) + c(z-z_{0} )\\ &=0. \end{aligned}$$

We can also write the above equation of the plane as

$$ax+by+cz+d = 0,$$

where $d= -(ax_{0} + by_{0} + cz_{0}) .$

This does not quite work if one of $a, b, c$ is zero. In that case the vector is parallel to one of the coordinate planes. Say $c = 0$ then the vector is parallel to the $xy$-plane and the equation of the required plane is $a(x-x_{0}) + b(y-y_{0}) = 0$ which is of course a straight line in the $xy$ plane and $z$ is unrestricted. Similar arguments apply if two of $a, b, c$ are zero.

Another way to think of the equation of the plane is as a flattened parallelepiped. A flattened parallelepiped, made of three vectors $\vec{a} = \left \langle x_{1}, y_{1}, z_1 \right \rangle , \vec{b} = \left \langle x_2, y_2, z_2 \right \rangle, \vec{c} = \left \langle x_3, y_3, z_3 \right \rangle$, has volume 0. We can use the scalar triple product to compute this volume:

$$0 = \vec{a} \cdot \big(\vec{b} \times \vec{c}\big),$$

where $\big(\vec{b} \times \vec{c}\big)$ gives the vector that is normal to the plane.

Let's say that the endpoints of $\big(\vec{b} \times \vec{c}\big)$ are $( x, y, z )$ and $(x_0, y_0, z_0 )$ and the components of $\vec{a}$ are $\left \langle a, b, c \right \rangle$. Then by taking the dot product, we get the equation of a plane, which is

$$0 = a(x-x_0) + b(y-y_0) + c(z-z_0).$$

Here is a problem to try:

The equation of a plane which is parallel to each of the $xy$-, $yz$-, and $zx$-planes and going through a point $A=(a,b,c)$ is determined as follows:

1) The equation of the plane which is parallel to the $xy$-plane is $z=c .$
2) The equation of the plane which is parallel to the $yz$-plane is $x=a .$
3) The equation of the plane which is parallel to the $zx$-plane is $y=b.$

Here is an example based on the above:

What is the equation of the plane which passes through the point $B=(4,1,0)$ and is parallel to the $yz$-plane?

---

Since the $x$-coordinate of $B$ is 4, the equation of the plane passing through $B$ parallel to the $yz$-plane is

$$x=4. \ _\square$$

Try the following problem:

If we know the normal vector of a plane and a point passing through the plane, the equation of the plane is established.

Thus, the equation of a plane through a point $A=(x_{1}, y_{1}, z_{1} )$ whose normal vector is $\overrightarrow{n} = (a,b,c)$ is

$$a(x-x_{1}) + b(y-y_{1}) + c(z-z_{1}) = 0 .$$

Check out the following examples:

If a plane is passing through the point $A=(1,3,2)$ and has normal vector $\overrightarrow{n} = (3,2,5),$ then what is the equation of the plane?

---

The equation of the plane which passes through $A=(1,3,2)$ and has normal vector $\overrightarrow{n} = (3,2,5)$ is

$$\begin{aligned} 3(x-1) + 2(y-3) + 5(z-2) &= 0 \\ 3x - 3 + 2y - 6 + 5z - 10 &= 0 \\ 3x + 2y + 5z - 19 &=0. \ _\square \end{aligned}$$

If a plane is passing through the point $A=(5,6,2)$ and has normal vector $\overrightarrow{n} = (-1,3,-7),$ then what is the equation of the plane?

---

The equation of the plane which passes through the point $A=(5,6,2)$ and has normal vector $\overrightarrow{n} = (-1,3,-7)$ is

$$\begin{aligned} -1(x-5) + 3(y-6) -7(z-2) &= 0 \\ -x+5+3y-18-7z+14 &= 0 \\ -x+3y-7z+1 &=0. \ _\square \end{aligned}$$

Try the following problem:

When we know three points on a plane, we can find the equation of the plane by solving simultaneous equations.

Let $ax+by+cz+d=0$ be the equation of a plane on which there are the following three points: $A=(1,0,2), B=(2,1,1),$ and $C=(-1,2,1).$ Then the equation of the plane is established as follows:

We already have the equation of the plane with 4 unknown constants:

$$ax + by + cz +d = 0. \qquad (1)$$

We also get the following 3 equations by substituting the coordinates of $A, B,$ and $C$ into $(1):$

$$\begin{aligned} a \cdot 1 + b \cdot 0 + c \cdot 2 + d &= 0 \\ a \cdot 2 + b \cdot 1 + c \cdot 1 + d &= 0 \\ a \cdot (-1) + b \cdot 2 + c \cdot 1 +d &= 0, \end{aligned}$$

which gives $b=3a, c=4a, d=-9a. \qquad (2)$

Substituting $(2)$ into $(1) ,$ we have

$$\begin{aligned} ax + 3ay + 4az -9a &= 0 \\ x + 3y + 4z - 9 &=0. \end{aligned}$$

Hence, the equation of the plane passing through the three points $A=(1,0,2), B=(2,1,1),$ and $C=(-1,2,1)$ is

$$x + 3y + 4z - 9 =0 .$$

Using this method, we can find the equation of a plane if we know three points. Here are a couple of examples:

If a plane is passing through the three points $A=(0,0,2), B=(1,0,1),$ and $C=(3,1,1) ,$ then what is equation of the plane?

---

Let the equation of the plane be $ax+by+cz+d=0. \qquad (1)$

Then since this plane includes the three points $A=(0,0,2), B=(1,0,1),$ and $C=(3,1,1) ,$ we have

$$\begin{aligned} a \cdot 0 + b \cdot 0 + c \cdot 2 + d &= 0 \\ a \cdot 1 + b \cdot 0 + c \cdot 1 + d &= 0 \\ a \cdot 3 + b \cdot 1 + c \cdot 1 +d &= 0, \end{aligned}$$

which gives $b=-2a, c=a, d=-2a. \qquad (2)$

Substituting $(2)$ into $(1) ,$ we have

$$\begin{aligned} ax + -2ay + az -2a &= 0 \\ x -2y + z - 2 &=0. \end{aligned}$$

Hence, the equation of the plane passing through the three points $A=(0,0,2), B=(1,0,1)$ and $C=(3,1,1)$ is

$$x -2y + z - 2 =0. \ _\square$$

If a plane is passing through the three points $A=(3,1,2), B=(6,1,2),$ and $C=(0,2,0) ,$ then what is the equation of the plane?

---

Let the equation of the plane be $ax+by+cz+d=0. \qquad (1)$

Then since this plane includes the three points $A=(0,0,2), B=(1,0,1),$ and $C=(3,1,1) ,$ we have

$$\begin{aligned} a \cdot 3 + b \cdot 1 + c \cdot 2 + d &= 0 \\ a \cdot 6 + b \cdot 1 + c \cdot 2 + d &= 0 \\ a \cdot 0 + b \cdot 2 + c \cdot 0 +d &= 0, \end{aligned}$$

which gives $a=0, c=\frac{1}{2}b, d=-2b . \qquad (2)$

Substituting $(2)$ into $(1) ,$ we have

$$\begin{aligned} 0x + -by + \frac{1}{2}bz -2b &= 0 \\ x -y + \frac{1}{2}z - 2 &=0 \\ 2x - 2y +z-4 &=0. \end{aligned}$$

Hence, the equation of the plane passing through the three points $A=(0,0,2), B=(1,0,1),$ and $C=(3,1,1)$ is

$$2x - 2y +z-4 =0. \ _\square$$

Try the following problem:

This section is dedicated to improve your problem-solving skills through several problems to try.

• 3D Coordinate Geometry - Parallel Planes

• 3D Coordinate Geometry - Perpendicular Planes

• 3D Coordinate Geometry - Intersection of Planes

• 3D Coordinate Geometry - Skew Lines