Analyzing Elastic Collisions
A perfectly elastic collision is one in which conservation of energy holds, in addition to conservation of momentum. As a result of energy's conservation, no sound, light, or permanent deformation occurs. As perfectly elastic collisions are ideal, they rarely appear in nature, but many collisions can be approximated as perfectly elastic.
 Newton's cradle exhibits nearly perfect elastic collisions, which is why it (ideally) swings back and forth in perpetuity. [1]
Newton's cradle exhibits nearly perfect elastic collisions, which is why it (ideally) swings back and forth in perpetuity. [1]
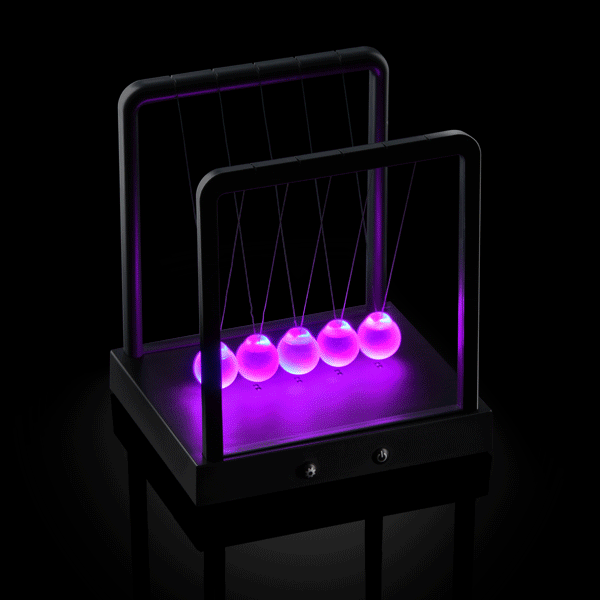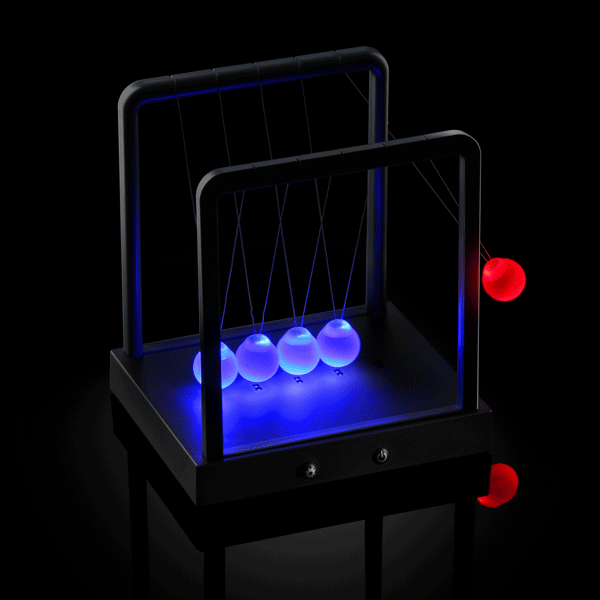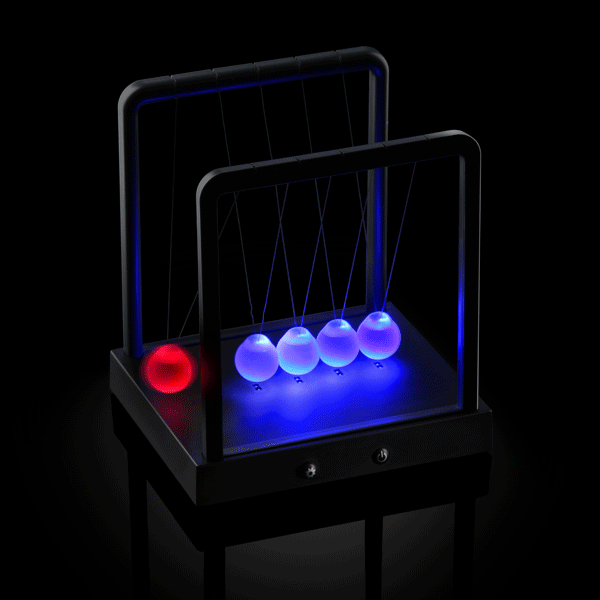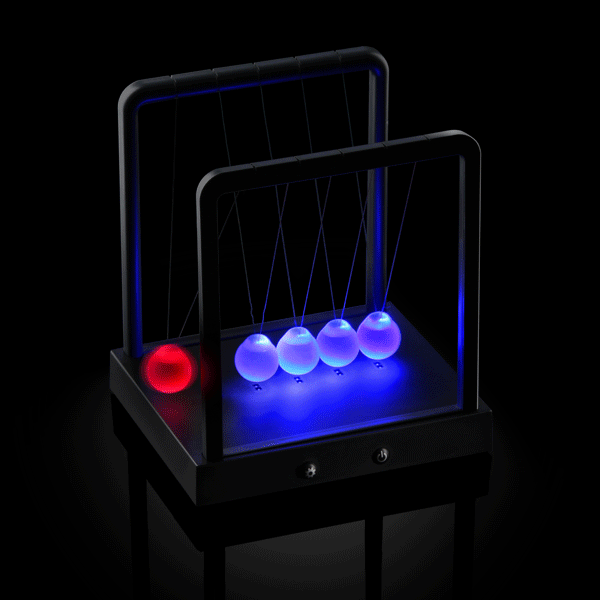
The gif given above demonstrates the working of a Newton’s cradle.
What does this amazing science toy explain to us?
Try my World of Physics to solve many problems like this one.
Collision in Slow Motion
To understand a collision, consider the following example:

In this case, a block of mass \({m_1}\) is coming towards a stationary mass \({m_2}\) with speed \(u\). A spring of spring constant \(k\) is attached to mass \({m_2}\) and the ground is frictionless. Now, when the moving block hits the spring, it tries to compress the spring. As the spring is compressed, it starts applying force on both the blocks. Due to this force, block \({m_1}\) is retarded and block \({m_2}\) is accelerated.
What can be said about the momentum of the system? Will it be conserved?
According to conservation of linear momentum, if net external force in any direction is zero, then the total momentum of the system is conserved. As the ground is smooth and the forces involved in the collision are internal forces which will be canceled in pairs, there is no force left in the horizontal direction and the momentum of the system of two blocks will be conserved.
In view of energies, the kinetic energy of block \({m_1}\) decreases and the potential energy and kinetic energy of \({m_2}\) increases. The speed of block \({m_1}\) will keep decreasing until its speed is greater than that of block \({m_2}\). When the speeds of the blocks become equal, the compression in spring is at its maximum. Beyond this point, the speed of block \({m_1}\) further decreases and the speed of block \({m_2}\) increases. Thus block \({m_2}\) starts moving faster and the spring starts recovering from compression.
Eventually, the spring gains its natural length and all the energy stored in the spring during the collision is restored back into the kinetic energy of the block. After the process of collision is over, part of kinetic energy of block \({m_1}\) is transferred to \({m_2}\). As the spring is considered ideal and perfectly elastic, no loss of kinetic energy eventually occurs. The kinetic energy during the collisions goes into the deformation energy, but due to perfect elastic nature of the spring, deformations are perfectly recovered and all the kinetic energy is recovered. Such a collision is called an elastic collision.
If the spring is not perfectly elastic and converts some of the potential energy into heat energy, then the final kinetic energy of the system will be less than the initial kinetic energy of the system. Such a collision is called an inelastic collision.
Elastic Collisions
An elastic collision is a collision in which colliding objects are perfectly elastic and the deformations occurring during collisions are fully recovered. Thus the kinetic energy of the colliding objects before collision equals the total kinetic energy after collision. \(_\square\)
Consider the diagram shown below. It shows a collision between two moving objects on a frictionless ground.

Here, \({u_1}\) and \({u_2}\) are initial velocities of blocks \({m_1}\) and \({m_2}\), respectively, and \({v_1}\) and \({v_2}\) are their corresponding final velocities after the collision.
The two objects are traveling along the same line and hit head-on with each other. The objects are perfectly elastic and the ground is smooth.
As there is no net external force in horizontal direction, linear momentum is conserved in horizontal direction:
\[{m_1}{u_1} + {m_2}{u_2} = {m_1}{v_1} + {m_2}{v_2}. \qquad (1)\]
Since the collision is perfectly elastic, the kinetic energy during the collision equals the kinetic energy after the collision:
\[\frac{1}{2}{m_1}u_1^2 + \frac{1}{2}{m_2}u_{_2}^2 = \frac{1}{2}{m_1}v_{_1}^2 + \frac{1}{2}{m_2}v_{_2}^2. \qquad (2)\]
Solving these two simultaneous equations, we get \[\begin{align} {v_1} &= \left( {\frac{{{m_1} - {m_2}}}{{{m_1} + {m_2}}}} \right)\,{u_1} + \frac{{2{m_2}{u_2}}}{{{m_1} + {m_2}}}\\ {v_2} &= \left( {\frac{{{m_2} - {m_1}}}{{{m_1} + {m_2}}}} \right)\,{u_2} + \frac{{2{m_1}{u_1}}}{{{m_1} + {m_2}}}. \end{align}\]
The velocity of approach is the rate at which the distance between the colliding objects is decreasing. In the above case as \({m_1}\) is approaching and \({m_2}\) is receding, the velocity of approach is \({u_1} - {u_2}\).
The velocity of separation is the rate at which the distance between the colliding objects (after the collision) is increasing. In the above case, as \({m_1}\) is receding and \({m_2}\) is still approaching after the collision, the velocity of separation is \({v_2} - {v_1}\).
On solving the equations (1) and (2), we can also get
\[{u_1} - {u_2} = {v_2} - {v_1},\]
where the term \({u_1} - {u_2}\) is called the velocity of approach and \({v_2} - {v_1}\) is called the velocity of separation.
Important points regarding elastic collision
If a perfectly elastic ball collides with a fixed surface, it rebounds with the same speed. A fixed surface can be treated as an infinite mass object with zero speed. Thus putting \({m_2} \to \infty \) and \({u_2}=0\) in the formula \[{v_1} = \mathop {\lim }\limits_{{m_2} \to \infty } \left[ {\left( {\frac{{{m_1} - {m_2}}}{{{m_1} + {m_2}}}} \right)\,{u_1} + \frac{{2{m_2}{u_2}}}{{{m_1} + {m_2}}}} \right],\] we get \({v_1} = - {u_1} .\)
If a perfectly elastic collision takes place between two objects of equal masses, then after the collision their velocities will exchange. That means the initial velocity of the first block will be the final velocity of the second block and vice versa. Thus, for the equal mass, we put \({m_1} = {m_2} \) in the formula \[{v_1} = \left( {\frac{{{m_1} - {m_2}}}{{{m_1} + {m_2}}}} \right)\,{u_1} + \frac{{2{m_2}{u_2}}}{{{m_1} + {m_2}}}\] and obtain \[{v_1} = {u_2}\] and \[{v_2} = {u_1}.\]
A block of mass \(m\) moving with horizontal speed 6 m/sec collides with a block of mass \(M\) moving with speed 4 m/s in the same direction. The ground is smooth. If \(m<<M,\) then for one-dimensional elastic collision find the speed of mass \(m\) after collision.

If \({v_1}\) is the final speed of mass \(m,\) then
\[{v_1} = \left( {\frac{{m - M}}{{m + M}}} \right)\,{u_1} + \frac{{2M{u_2}}}{{m + M}}.\]
As \(m<<M,\) we get
\[{v_1} = - {u_1} + 2{u_2}= - \,6 + 2(4) = 2 ~(\text{m/s}).\]
That is, the lighter particle will move in the original direction with a speed of 2 m/s. \(_\square\)
Six identical balls are lined in a straight groove made on a horizontal, frictionless surface, as shown below. Two similar balls each moving with velocity \(v\) collide with the row of 6 balls from left.
What will happen after the collision, if the collisions are perfectly elastic?

References
- Toussaint, D. Newtons cradle animation book 2. Retrieved May 25, 2016, from https://commons.wikimedia.org/wiki/File:Newtons_cradle_animation_book_2.gif