Specific Heat
Specific heat is a very important aspect of physics. To understand this, we must get to know what actually is heat. There are many day-to-day activities which involve the use of heat, but we don't realize its importance. Its uses range from simply rubbing our hands to keep ourselves warm to its use in complex machinery. The concept of specific heat, though it looks very difficult, can be dealt with very easily. So, let's explore the unique world of thermodynamics!
Heat
Heat is a form of energy. As discussed earlier, while we rub our hands, the energy spent in overcoming friction is converted into heat energy, or simply the mechanical energy is converted to heat. Matter contains heat in the form of kinetic energy and potential energy. The total mechanical energy, i.e. potential energy + kinetic energy, is hence called thermal energy.
The thermal energy in a particular body is proportional to the amount of inter-molecular vibration. This means that as the vibration increases the thermal energy also increases. So, hot bodies have more thermal energy than cold bodies. We know that when a hot body and a cold body are kept in contact with each other, the vibrations travel from the hot body to the cold.
Let's now define heat considering all the conditions:
Heat is a type of mechanical energy, which is stored in matter in the form of vibrational energy. It is the amount of thermal energy that flows from a hot body to a cold body. \(_\square\)SI unit: Joule
Thermal Equilibrium
We know that heat moves from a hot body to a cold body. When do you think will the transfer of heat stop? Or is it a never-ending process?
The transfer of heat energy stops when both objects get equally heated, i.e. the flow of heat continues till the temperatures are equalized.
When two bodies are undergoing the process of transfer of heat, the flow will continue if at least one of the body is hotter than the other. So, when the temperatures of both objects are equalized, the flow stops. In this situation the two bodies are said to be in thermal equilibrium. \(_\square\)
It obeys the zeroth law of thermodynamics.
Temperature
We have been talking about heat until now. But how do we measure this heat?
Temperature can be termed as a quantitative measure of the magnitude of hotness of a body. Or by using the concept of heat we define it as the average kinetic energy per molecule of a particular substance. \(_\square\)
Temperature is measured using various scales, and the most commonly used ones are the Celsius and Fahrenheit scales. Most of us might be familiar with the formulas of conversion between the two scales, which are as follows:
\[\begin{align} \text C&=\text F-32\times \dfrac59 \\ \text F&=\dfrac95 \times \text C+32, \end{align}\]
where \(C\) represents Celsius and \(F\) represents Fahrenheit. Another scale is the Kelvin scale whose conversion formula is as follows:
\[\text K=\text C+273.16,\]
where \(K\) represents Kelvin. But to make calculations simple, the value added is only \(273.\) Kelvin scale is taken as the \(\text{SI}\) unit for temperature. Another scale which only few of us are familiar with is the Newton scale, in which the boiling point is \(33\text{ N},\) so the conversion formula is
\[\dfrac{\text{N}}{0.33}=\text C.\]
Specific Heat
Now, as we have understood the concepts of heat and temperature, we can proceed to the main topic specific heat. We know that there are many factors affecting the increase in temperature per unit time for various bodies. Let's consider the following cases:
Case 1: There are two containers \(A\) and \(B,\) each containing an equal amount of water. Let us suppose that \(A\) is given more heat than \(B,\) then we see that the temperature rises faster in \(A.\) So we conclude that heat energy \(\text Q\) is directly proportional to the rise in temperature \(\Delta \text T\), or
\[\text Q\propto \Delta \text T.\]
Case 2: There are two containers \(A\) and \(B,\) where \(B\) contains more water than \(A\) and they are given an equal amount of heat. Then we see that the temperature rises faster in \(A.\) So we conclude that as the mass \(m\) of a body increases, the heat energy required also increases:
\[\text Q\propto m.\]
Case 3: For the last time, let \(A\) contain kerosene, and \(B\) an equal amount of water. Let them each be provided with an equal amount of heat, then we see that kerosene gets heated up earlier. So we conclude that the heat energy required is dependent on the nature of the substance, too.
Now, from cases (1) and (2), we have \(Q\propto \Delta T\) and \(Q\propto m\), which implies
\[\begin{align} \text Q&\propto m \Delta \text T \\ \text Q&=\text Cm\Delta \text T, \end{align}\]
where \(\text C\) is a constant of proportionality. This is called the specific heat capacity, whose \(\text{SI}\) unit is \(\text{J kg}^{-1} \text{K}^{-1}.\)
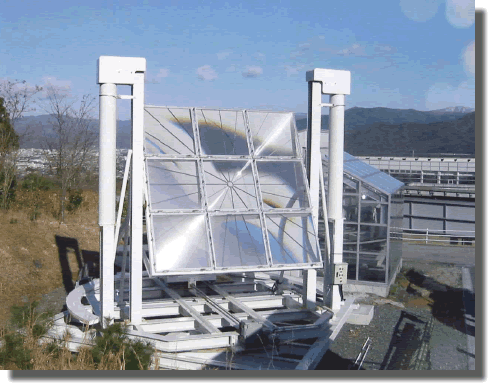
For how many seconds would you need to focus the Fresnel lens on a penny in order to melt the entire coin?
Details and assumptions:
- A penny weighs \(2.5 \text{ g}\) and is made of \(100\)% \(\text{Cu}.\)
- The penny starts out at \(25^\circ\text{C}\) and melts at \(1085^\circ\text{C}.\)
- \(\text{Cu}\)'s heat of melting is \(176 \text{ kJ}/\text{kg}\) and its specific heat is \(0.386\text{ kJ}/\text{kg}\cdot\text{K}.\)
- The area of the lens is \(0.2 \text{ m}^2,\) the power of the sun is \(1370\text{ W/m}^2,\) and \(100\)% of the energy goes into heating the coin.
Now, given that you are cooling it down to \(150^\circ\text{C}\), calculate the amount of heat (in Joules) given out during the process of cooling.
Details and assumptions:
- The specific heat capacity of aluminium is \(\text{C}_\text{Aluminum}=900 \text{ J}/\text{kg K}.\)
- Ignore the heat energy lost to the atmosphere.