Gauss's law
Gauss's law states that any charge \(q\) can be thought to give rise to a definite quantity of flux through any enclosing surface. Physically, we might think of any source of light, such as a lightbulb, or the Sun, which has a definite rating of power which it emits in all directions. No matter what shape of enclosing surface we trap it in, and no matter how near or far the light source is from the surface, the enclosing surface will receive the same amount of energy per unit time \(P_0\).
In a similar way, a distribution of total charge \(\sum_i q_i\) will give rise to an invariant amount of flux through any enclosing surface, which is defined to be the sum over all infinitesimal patches of surface \(dA\), of the electric field component perpendicular to the surface. Although not always a practical tool, in situations where geometrical symmetries can be exploited, Gauss's law is an incredibly powerful tool to quickly calculate electric fields. Analogous laws hold for other inverse square laws, e.g. Newtonian gravity.
Contents
Theorem Statement
Gauss's law of electric flux states that the net electric flux through any closed surface is directly proportional to the charge enclosed by the surface:
\[ \Phi_E = \frac{q_\text{tot}}{\varepsilon_0} .\]
It can be shown that this statement is equivalent to Coulomb's inverse square law, i.e. any force for which the inverse square law holds will also satisfy Gauss's law, and any force which satisfies Gauss's law will behave like an inverse square. Gauss's law is one of the four Maxwell equations for electrodynamics and describes an important property of electric fields. If one day magnetic monopoles are shown to exist, then Maxwell's equations would require slight modification, for one to show that magnetic fields can have divergence, i.e. \(\nabla \cdot B \sim \rho_m\). Cosmological theories do, however, predict that magnetic monopoles did exist at the beginning of the universe but collapsed due to their high instabilities.
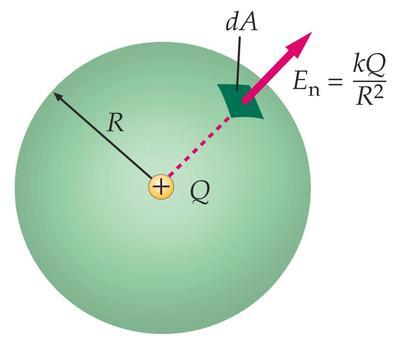
Closed Surfaces
A closed surface is a surface that is compact and without boundary. In other words, a closed surface is one that divides space (excluding itself) into two disjoint parts, an exterior and an interior. Some simple examples of closed surfaces include intact bubbles, Dyson spheres, or the enclosure one would be inside of if they were to get into a sleeping bag and sew the opening shut.
Gauss's law is a very powerful method to determine the electric field due to a distribution of charges. The mathematical expression for Gauss's law is
\[ \int_{S} \vec{E} \cdot \vec{dA}=\frac{Q_{enc}}{\epsilon_0} ,\]
where \(S\) is a surface, \(\vec{E}\) is the electric field vector, \(\vec{dA}\) is the infinitesimal area element, \(Q_{enc}\) is the charge enclosed by \(S,\) and \(\epsilon_0\) is a constant.
In order to apply Gauss's law, we need to understand what each of the parts of this expression means. This set of problems will help you understand each of the components. Let's start with \(S\). You may be more familiar with integrals as the limit of a sum of a function over a line interval, which gives the "area under the curve." An integral over a surface of a function is just the sum of that function over all the points on the surface.
The surface in Gauss's law is a closed two-dimensional surface, such as the surface of a sphere or the surface of a cube. A closed surface is a surface that divides space into an inside and an outside, whereby dividing we mean there is no path that goes from inside to outside that does not penetrate the surface. Consider the surface \(S\) of the objects below. For which of the objects is \(S\) a closed surface?
Flux of a Vector Field
Loosely spoken, the flux of a field through a surface is the net flow through it. We develop this intuition in the example below.
Think of this analogy:
Assume you fit a cotton membrane in the middle of a pipe through which water is flowing. What is the flow of water through the membrane?
Of course, the answer would be the average normal component of the velocity times the area of the membrane.
This is what we call the flux through the membrane!
Why do we take the normal component? Because the alignment of the membrane with the direction of the flow matters. What if both the membrane and the flow are aligned horizontally?
\[ \Phi = \oint_{\mathcal{S}} \overrightarrow{E} \cdot d \overrightarrow{A}.\]
Divergence of a Vector Field
Divergence of a vector field at a point is the magnitude of the field's source or sink at that point. Of course, this is the same as stating that the divergence represents the volume density of the outward flux of a vector field from an infinitesimal volume around a given point.
Formally stated, the above translates to
The divergence of a vector field \(\overrightarrow{E}\) at a point \(p\) is defined as the limit of the net flow of \(\overrightarrow{E}\) across the smooth boundary of a three-dimensional region \(V\) divided by the volume of \(V\) as \(V\) shrinks to \(p\):
\[\operatorname{div}\,\overrightarrow{E}(p) = \lim_{V \rightarrow \{p\}} \iint_{S(V)} {\overrightarrow{E}\cdot\widehat{n} \over |V| } \; dS, \]
where \(|V|\) is the volume of \(V\), \(S(V)\) is the boundary of \(V\), and the integral is a surface integral with \(\widehat{n}\) being the outward unit normal to that surface.
Application in Cartesian coordinates:
If there is a vector field such that
\[ \overrightarrow{E}(x \widehat{i} + y \widehat{j} + z \widehat{k}) = u \widehat{i} + v \widehat{j} + w \widehat{k}, \]
then
\[ \operatorname{div}\,\overrightarrow{E} = \nabla \cdot \overrightarrow{E} = \frac{\partial{u}}{\partial{x}}+\frac{\partial{v}}{\partial{y}}+\frac{\partial{w}}{\partial{z}} .\]
Gauss's Law for Electric Field
Integral Form
If \(S\) is a closed surface in which a charge \(Q\) is enclosed, then the flux \(\Phi_E\) through \(S\) is given by
\[ \Phi_E = \oint_{\mathcal{S}} \overrightarrow{E} \cdot d \overrightarrow{A} =\frac{Q}{\varepsilon_0}. \]
Differential Form
If \( \rho \left(\overrightarrow{r}\right)\) is the volume density of the charge at \(\overrightarrow{r}\), then the divergence of the electric field \(\overrightarrow{E}\) at \(\overrightarrow{r}\) is
\[ \nabla \cdot \overrightarrow{E}\left(\overrightarrow{r}\right) = \frac{\rho \left(\overrightarrow{r}\right) }{\varepsilon_0} .\]
The above discussion on flux and divergence should make it clear why these two forms are equivalent. Nevertheless, this equivalence comes from Gauss's theorem or the divergence theorem.
Gauss Law for other Important Fields
A similar statement such as the electric Gauss law could be made for several other fields. Here is a table of such expressions where symbols have their usual meanings.
| Field | Integral Form | Differential Form |
| Gravitational\(\hspace{20mm}\) | \(\oint \overrightarrow{g} \cdot d \overrightarrow{A} = -4 \pi G M\)\(\hspace{20mm}\) | \( \nabla \cdot \overrightarrow{g} =-4 \pi G \rho \) |
| Magnetic | \(\oint \overrightarrow{B} \cdot d \overrightarrow{A} = 0\) | \(\nabla \cdot \overrightarrow{B} = 0 \) |
Equivalence with Coulomb's Law
Deriving Coulomb's from Gauss:
Consider a charge \(Q\) and a sphere of radius \(r\).
By Gauss's law the flux of the field from the sphere is \(\frac{Q}{\varepsilon},\) which is equal to \(4 \pi r^2 |E|, \) so
\[ 4 \pi r^2 |E| = \frac{Q}{\varepsilon} \Rightarrow |E| = \frac{Q}{4 \pi r^2 \varepsilon}. \]
So, placing a test charge \(q\) in the field which does not disturb it results in a force of
\[ |F| = q |E| = q \frac{Q}{4 \pi r^2 \varepsilon}, \]
which is the Coulomb's law.
Please read John Muradeli's note for a better explanation of the above proof.
Deriving Gauss from Coulomb's:
We start with Coulomb's law for a single point charge \(E = \frac{\gamma}{r^2}\), where \(\gamma = \frac{q}{4\pi \varepsilon_0}\).
Now, we integrate the electric field over a closed spherical surface encasing the charge:
\[\begin{align} \oint E \cdot \hat{n}\, dA &= \oint \frac{\gamma}{r^2} \, dA \\ &= \frac{\gamma}{r^2}\oint\, dA \\ &= \frac{\gamma}{r^2} 4\pi r^2 \\ &= 4\pi\gamma \\ &= \frac{q}{\varepsilon_0}, \end{align}\]
which is Gauss's law, as desired. \(_\square\).
Note: The proof offered only works for spherical surfaces; a more general proof ends up using vector calculus and Green's/Delta functions. Feel free to take a shot at the general case.
Applications
Gauss's law is a powerful statement about inverse square fields. Not just in problem-solving, it has also found its place in the four Maxwell equations as well as in gravity.
Suppose that an infinitely long straight current-carrying wire has a uniform linear charge density \(\lambda.\) Let this wire be on the \(y\)-axis of the \(xy\)-plane, and let \(x > 0\) be the distance between the \(y\)-axis and a point \(P\) on the \(xy\)-plane. What is the strength of the electric field at the point \(P?\)
Note: \(\epsilon_0 \) in the choices below denotes the electric constant.
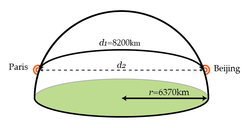
The gravity train is a hypothetical idea proposed by Robert Hooke (he of Hooke's law fame) to Isaac Newton in the 1600's. It consists of a simple idea that's hard to implement in practice. Dig a tunnel that runs straight through Earth between two points on the surface. If you can figure out how to remove friction and lower air resistance, you now have a mechanism for extremely efficient and rapid travel between widely separated points. Simply drop something into the tunnel at one end. Gravity will initially pull it downwards through the tunnel, eventually reaching high speeds. Once the object is halfway through the tunnel, gravity will now slow it back down, so you can retrieve the object easily on the other side.
While there are obvious engineering impracticalities, it winds up being pretty amazing how fast a gravity train can get things from point to point without needing fuel. So, let's say we wanted to go from Beijing to Paris using such a gravity train. How long does the trip take assuming a frictionless and drag-free train to the nearest minute?
Earth can be modeled as a sphere of uniform density, with total mass \(6 \times 10^{24}~\mbox{kg}\) and radius \(6370~\mbox{km}\). The shortest distance from Beijing to Paris on the surface of Earth is approximately \(8200~\mbox{km}\).
Details and Assumptions:
- Newton's constant is \(6.67 \times 10^{-11}~\mbox{N m}^2/\mbox{kg}^2\).
- The mass of the Earth is \(6 \times 10^{24}~\mbox{kg}\).
See David's Set for a tour of Gauss's theorem and its applications.