Ohm's Law (Microscopic Interpretation)
Microscopically, Ohm's law is a statement about how application of an electric field to a conducting material leads to an electric current:
\[\vec{J} = \sigma \vec{E}.\]
In the above equation, \(\sigma\) is a constant called the conductivity of a material, \(\vec{E}\) is the applied electric field, and \(\vec{J}\) is the electric current density at a point. The conductivity of a material therefore measures the extent to which electrons in the material respond to an applied field.
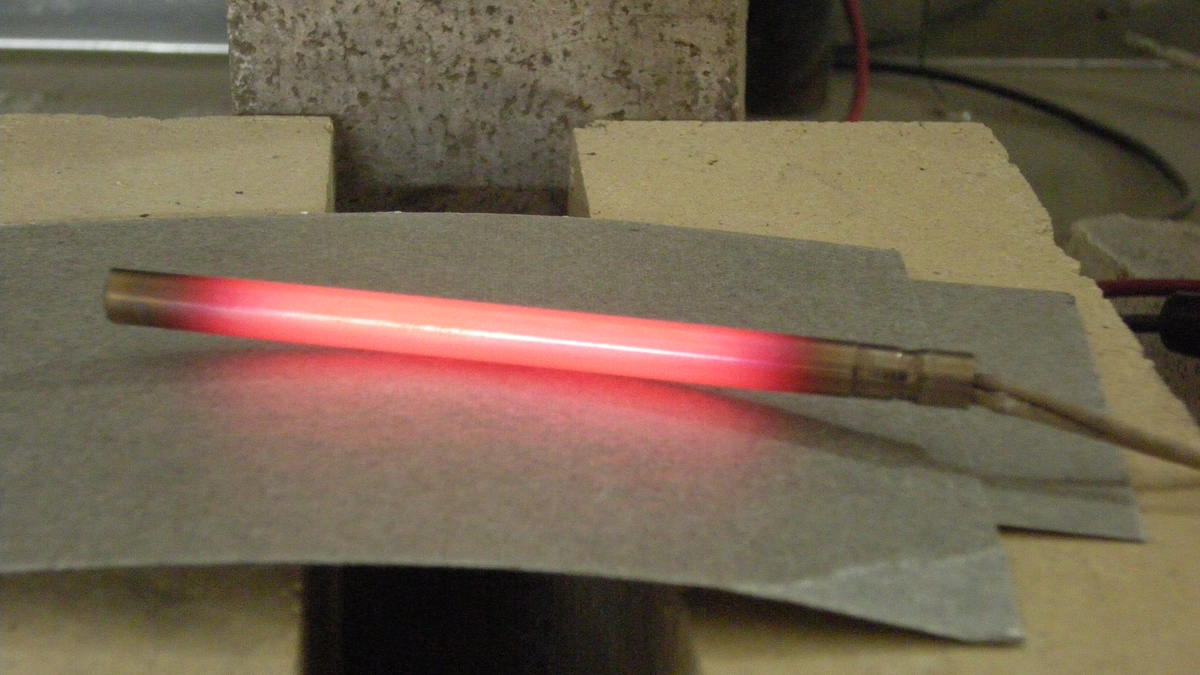 Electric current running through a cartridge heater, causing a red-hot glow due to low conductivity / high resistance. [1]
Electric current running through a cartridge heater, causing a red-hot glow due to low conductivity / high resistance. [1]
By considering the dynamics of electrons in conducting materials, it is possible to understand the different electrical properties of different materials. For instance, the change in electron dynamics leads to the distinction between superconductors, conductors, semiconductors, and insulators, all of which have a vast array of technological uses.
Contents
Application of Ohm's Law
Ohm's law relates the current density in a conductor to the applied electric field, by the formula \(J = \sigma E\) given above. The conductivity \(\sigma\) is given by the formula
\[\sigma = n_e e^2 \frac{\tau}{m_e}\]
with \(n_e\) the volume density of conduction electrons, \(e\) the electron charge, \(m_e\) the electron mass, and \(\tau\) the mean free time of the electrons, representing how long on average a conduction electron travels before interacting with the conductor. One also often works in terms of the quantity \(\rho = \frac{1}{\sigma}\), the resistivity.
Using this formula, the current density of electrons can be rewritten in terms of the average velocity of the electrons, often called the drift velocity:
\[\vec{J} = -en_e \bar{\vec{v}}.\]
For electron motion in a bar, the microscopic Ohm's law can be related to the macroscopic Ohm's law \(V=IR\). Note that current density is current per unit area \(J = \frac{I}{A}\). Similarly, an electric field is a voltage per unit length: \(E = \frac{V}{L}\). Combining the two, one finds
\[V = \left(\frac{L}{A\sigma}\right) I.\]
In a conducting bar of cross-sectional area \(A\) and length \(L\) with conductivity \(\sigma\), the resistance is therefore defined as
\[R = \frac{L}{A\sigma} = \frac{\rho L}{A}.\]
In complicated materials where the conductivity changes over the length of the conductor, the resistance is found by treating everything above as an infinitesimal quantity and integrating.
A strange metal bar of cross-sectional area \(A\) stretches from \(x=1\) to \(x=L\) with resistivity \(\rho(x) = \frac{1}{x}\). Compute the resistance of this bar.
The resistance of a small piece of the bar is
\[dR = \frac{\rho(x) dx}{A}.\]
Integrating, one finds
\[R = \int_1^L \frac{1}{xA} dx = \frac{\log(L)}{A}.\ _\square\]
A pure germanium wire of resistivity \(\rho = 1.2 \times 10^{-3} \:\Omega\cdot \text{m}\) and length \(10 \text{ cm}\) is connected to either terminal of a \(9 \text{ V}\) battery. Suppose that the total mass of the wire is \(20 \text{ g}\) and that only one electron conducts per germanium atom. Find the drift velocity of the electrons in the wire.
The magnitude of the current density as given above is
\[J = en_e v,\]
where \(v\) is the drift velocity. There are two things to compute: the density \(n_e\) of conducting electrons and the current density \(J\).
The current density is found from
\[J = \sigma E = \frac{V}{\rho L},\]
where \(L\) is the total length of wire, which is given.
The number of conducting electrons can be computed from the total number of germanium atoms, since each atom only provides one conducting electron. The number of germanium atoms can be computed from the total mass: since germanium weighs \(72.3 \text{ g}\) per mole, there are
\[20 \text{ g} \times \frac{1 \text{ mole}}{72.3 \text{ g}} \times \frac{6.022\times 10^{23} \text{ electrons}}{1 \text{ mole}} = 1.67 \times 10^{23} \text{ electrons}.\]
The factor \(n_e\) gives the density of conducting electrons, so we need the total volume. In \(20 \text{ g}\) of germanium, the volume is \(\big(\)from the density \(5.5 \text{ g}/\text{cm}^3\) of germanium\(\big)\)
\[V = \frac{20 \text{ g}}{5.5 \text{ g}/\text{cm}^3} = 3.64 \text{ cm}^3,\]
so that the electron density is
\[n_e = \frac{1.67 \times 10^{23}}{ 3.64 \text{ cm}^3} = 4.59 \times 10^{22} \text{ cm}^{-3}.\]
The drift velocity is therefore
\[ \begin{align} v &= \frac{J}{en_e} \\&= \frac{V}{\rho L e n_e} \\ &= \frac{9 \text{ V}}{( 1.2 \times 10^{-3} \:\Omega\cdot \text{m})(10 \text{ cm})(1.6 \times 10^{-19} \text{ C}) (4.59 \times 10^{22} \text{ cm}^{-3} )} \\&= 1.02 \times 10^{-5} \text{ m}/\text{s} . \end{align} \]
This velocity is very slow! Most of the speed of electrical signals comes from the propagation of "holes" in charge through materials rather than actual physical charges. \(_\square\)
A copper wire of resistivity \(1.8 \times 10^{-8} \:\Omega\cdot\text{m}\) and length \(L = 1 \text{ m}\) is connected to either terminal of a \(1.5 \text{ V}\) battery. If the density of conduction electrons is \(3 \times 10^{29} \text{ m}^{-3}\), find the drift velocity of the conduction electrons in millimeters per second.
Electron Dynamics in Conductors
Above, the conductivity was defined via the formula
\[\sigma = n_e e^2 \frac{\tau}{m_e},\]
where \(\tau\) was some unknown timescale. One can determine this timescale by considering how this formula was derived: the change in momentum from applying an electric force over some period of time was computed two different ways. The total amount of time an electric force accelerates a conduction electron is the time until it scatters off an atom in the metal and loses energy. Thus \(\tau\) is the mean free time of an electron in a conductor. This means that \(\tau\) is the average time it takes a conduction electron to interact with an atom in the conductor and lose energy. In highly conductive materials, conduction electrons may be accelerated for a long time before interacting with the conductor, consistent with the above formula.
The mean free time may be related to the mean free path \(\lambda\) using the formula
\[\lambda = v \tau,\]
where \(v\) is the drift velocity of the electrons. That is, the mean free path is the total distance electrons tend to travel in the mean free time.
In some metal, the density of conduction electrons is \(n_e = 10^{30} \text{ m}^{-3}\), the drift velocity of electrons is \(10^{-6} \text{ m}/\text{s}\), and the resistivity of the metal is \(\rho = 10^{-3} \Omega \cdot \text{m}\). Compute the mean free path of the conduction electrons.
From the formula for the conductivity,
\[\frac{1}{\rho} = n_e e^2 \frac{\tau}{m_e} = n_e e^2 \frac{\lambda}{m_e v},\]
which rearranges to
\[\lambda = \frac{m_e v}{n_e \rho e^2}.\]
Plugging in for all quantities, one obtains the result
\[\lambda = 3.548 \times 10^{-26} \text{ m} .\ _\square\]
Using the fact that the magnitude of the current density is related to the drift velocity by
\[J = \sigma E = en_ev,\]
the drift velocity can be related to the conductivity in terms of the applied field by
\[\frac{v}{E} = \frac{\sigma}{en_e}.\]
The quantity on the left-hand side is called the electron drift mobility and is often written as
\[\mu = \frac{v}{E}.\]
Suppose the measured electron drift mobility in a metal is \(\mu = 12 \text{ cm}^2 \text{V}^{-1} \text{s}^{-1}\) and that the density of conduction electrons in the metal is \(2\times 10^{28} \text{ m}^{-3}\). What is the conductivity of this metal?
The conductivity is computed straightforwardly by rearranging the formula for electron drift mobility:
\[\sigma = en_e\mu = \big(1.6 \times 10^{-19} \text{ C}\big) \big(2\times 10^{28} \text{ m}^{-3}\big) \big(12 \text{ cm}^2 \text{V}^{-1} \text{s}^{-1}\big) = 3.86 \times 10^6 \text{ s}^3\text{A}^2 \text{kg}^{-1} \text{m}^{-3}.\ _\square\]
In some metal, the mean free time between interactions of the conduction electrons with the metal is \(\tau = 4 \times 10^{-13} \text{ s}\) and the drift velocity of the electrons is \(v = 5 \times 10^{-2} \text{ cm}/\text{s}\). What is the mean free path of the conduction electrons, in meters?
Derivation of Ohm's Law
In a conducting material, electrons are loosely bound to their constituent elements, and a small amount of energy (via an applied electric field) is sufficient to mobilize them, creating an electric current. Current is measured in amperes, where one ampere is equivalent to one Coulomb of charge per second.
If there is a volume density \(n_i\) of charges of charge \(q_i\) and velocity \(\vec{v}_i\), then the current density in the material is
\[\vec{J} = \sum_i n_i q_i \vec{v}_i.\]
This formula comes from dimensional analysis. The total current per unit area due to some type of charge is just the density of charges per unit area \(n_i q_i\) times the velocity of those charges \(\vec{v}_i\).
Since there are typically many charges in a material, it is often more useful to work in terms of the average velocity of charges. The average velocity of one particular type of charge (e.g. electrons of charge \(-e\)) is given in terms of the density of electrons \(n_e\) by
\[\bar{\vec{v}} = \frac{1}{n_e} \sum_i n_i \vec{v}_i.\]
The current density due to electrons can then be written as
\[\vec{J} = -en_e \bar{\vec{v}}.\]
In order to relate the current density to an applied electric field, it is useful to consider how the average velocity of electrons is related to the applied field. The change in momentum of an electron is equal to the impulse on it by the field: \(\Delta p = F\tau\). On one hand, the momentum of an electron will be given by \(\Delta p= m_e \bar{\vec{v}}\). On the other hand, the force on the electron is \(-e\vec{E}\). Thus we can write
\[m_e \bar{\vec{v}} = -e\vec{E} \tau \implies \vec{J} = \left(N_e e^2 \frac{\tau}{m_e}\right) \vec{E}.\]
Defining \(\sigma = n_e e^2 \frac{\tau}{m_e}\), Ohm's law is therefore derived from the microscopic motion of electrons in a conductor.
Ohm's law doesn't represent a fundamental law of nature. It states about the relationship that the resulting current \(I\) is proportional to the applied emf \(\mathbb{E}=IR\). Emf is \(\mathbb{E}=\oint E\cdot ds\). In Ohm's law, the resistivity \(\rho=\frac{AR}{L}\) is the property of materials only, not its dimensions. Alternatively, we consider conductivity \(\sigma=\frac{1}{\rho},\) and then the Ohm's law is defined as \(J=\sigma E\),\(J\) is the current density.
To get a proper derivation of Ohm's law, we need quantum mechanics and microscopic understanding. Whence we write \(E=-\nabla\phi\), then \(\mathfrak{E}=V\), the potential difference between two ends of the wire.
References
- Maxellator, . CC-3.0 Licensing. Retrieved from https://commons.wikimedia.org/wiki/File:Cartridge-heater-hot.jpg