Solve Linear Systems (Algebra)
A system of linear equations is a collection of linear equations which involve the same set of variables. As an example,
\[ \begin{align} x+2y & =2 \\ -x+y & =1 \end{align} \]
is a system of equations that has two variables \(x\) and \(y.\) The solution to a linear system is an assignment of numbers to the variables that satisfy every equation in the system. For a given system, we could have one solution, no solutions or infinitely many solutions.
Contents
Substitution Method
In this method, we
- Find a relation that isolates one of the variables by changing the subject.
- Substitute the relation into the other equation(s) to reduce the number of variables by 1.
- Repeat until we are left with a single variable, and solve for it.
- Substitute the solved value back into the relations.
- State the complete solution.
Let's follow the above steps to solve the following system of equations:
\[\begin{align} x+y &= 5 \\ x-y &= 3. \end{align}\]
Step 1: Isolate variable \(x\) using the second equation: \( x = 3 + y \).
Step 2. Substitute the relation into the other equation: \( (3 + y ) + y = 5 \Rightarrow 3 + 2y = 5 \).
Step 3. Repeat, and then solve. Since we now have only 1 variable, solve for it: \( 3 + 2y = 5 \Rightarrow y = \frac{5-3}{2} = 1 \).
Step 4. Substitute back into the relations: \( x = 3 + y = 3 + 1 = 4 \).
Step 5. State the complete solution: \( (x,y) = (4,1) \).
Solve the system of equations
\[ \begin{align} 2x + y &= 10\\ 2y &= 8 . \end{align}\]
In this example, because we are already given \( 2y = 8 \) in the second equation, we know that \( y = 4 \). Substituting this into the first equation, we get \( 2x + 4 = 10 \), and thus \( x = 3 \). \(_\square\)
Note: It matters which equation we use and which variable we isolate out. In this example, even if we obtained \( x = \frac{ 10 - y } { 2 } \) from the first equation, we find that we are unable to substitute it into the second equation as there is no \(x\) term.
Solve the system of equations
\[ \begin{align} 2x + y &= 4\\ 3x + 2y &= 7. \end{align}\]
Solving the first equation for \(y\) gives \(y=4-2x\). Substituting this into the second equation gives \[\begin{align} 3x + 2(-2x+4) &= 7\\ 3x -4x + 8 &= 7\\ -x & = -1\\ x & = 1. \end{align}\] Then substituting this value into the first equation, we have \[y=-2x + 4= -2(1) + 4 = -2 + 4 = 2.\] Therefore, the solution to the system of equations is \(x=1, y=2\). \(_\square\)
Using the process of substitution may not be the quickest nor the easiest approach for a given system of linear equations. However, we are always guaranteed to find the solution, if we work through the entire process. The word "system" indicates that the equations are to be considered collectively, rather than individually. Thus the solution must not lose validity for any of the equations. Select your options so that your calculations are simple and use any method that suits you.
\(x\) and \(y\) are real numbers that satisfy \(2x = 50\) and \(x + y = 40\). What is the value of \(y\)?
Elimination Method
The elimination method multiplies the given \(n\) equations with suitable constants so that when the modified equations are added, one of the variables is eliminated. Once this is done, the system will have effectively been reduced by one variable and one equation. This process is repeated until one variable and one equation remain (namely, the value of the variable). From there, the obtained value is substituted into the equation with 2 variables, allowing a solution to be found for the second variable. The process is repeated until the values of all \(n\) variables are found.
Method
- Find two equations that have the same variable. Multiply each equation by a number such that their coefficients are equal.
- Subtract the two equations.
- Repeat until we are left with a single variable, and solve for it.
- Substitute the solved value back into the original equations to solve for the remaining variables.
Let's follow the above steps to solve the following system of equations:
\[\begin{align} 3x - 4y &= 0 \\ 9x - 8 y &= 12. \end{align}\]
Step 1: Multiply each equation by a number such that the coefficients of a variable are the same.
Let's say that we want to eliminate the variable \(x\).
Multiply the first equation by 3 and the second equation by 1, and we obtain
\[ 9 x - 12 y = 0, 9 x - 8 y = 12. \]Step 2: Subtract the two equations:
\[\begin{align} 9x - 12y &= 0 \\ - (9x - 8 y &= 12) \\ \hline -4 y & = - 12. \end{align} \]Step 3: Repeat and solve.
We are already down to one variable. Solving it gives us \( y = \frac{ -12}{-4} = 3 \).Step 4: Substitute the solved value back into the relations.
Substituting into the first equation, we get \( 3x - 4 \times 3 = 0 \), or \( x = \frac{12}{3} = 4 \).
Solve the following system of equations: \[\begin{cases} 2x+y=7 \\ 4x-y=5. \end{cases}\]
System of Linear Equations - More Variables
When we have more variables to work with, we just have to remember to stick to a particular method, and keep on reducing the number of equations or variables.
We will solve the following system of equations using both approaches:
\[ \begin{align} x + 3y - z &= 6\\ 2x - y + 2z &= 1\\ 3x + 2y - z &= 2. \end{align}\]
Substitution method
We begin with the first of the above three equations:
- Step 1: The first equation gives \(x+3y-z=6 \Rightarrow x=6-3y+z\).
- Step 2: Substituting for \(x\) in the second equation, we obtain \[ \begin{align} 2(6-3y+z) - y + 2z &= 1\\ 12 - 6y + 2z - y + 2z &= 1\\ -7y + 4z &= -11. \qquad (1) \end{align}\] Substituting for \(x\) in the third equation, we obtain \[ \begin{align} 3(6-3y+z) + 2y - z & = 2\\ 18 - 9y + 3z + 2y - z &= 2\\ 2z - 7y &= -16. \qquad (2) \end{align}\]
- Step 3. We need to repeat until we have only one equation.
Now, we begin with \((1)\) of the two equations \((1)\) and \((2)\) above:
- Step 1: The equation \((1)\) gives us \( y = \frac{ 4z+11}{7} \).
- Step 2: Substituting for \(y\) in \((2)\) gives \[ \begin{align} 2z - 7 \frac{ 4z+11}{7}&= -16\\ 2z - ( 4z + 11 ) & = - 16 \\ -2z & = -5. \end{align}\]
- Step 3: We are now down to one equation. Solving it gives us \( z = \frac{-5}{-2} = \frac{5}{2} \).
- Step 4: Substitute \( z = \frac{5}{2} \) into \( y = \frac{ 4z + 11} { 7} \) to obtain \[ y = \frac{ 4z+ 11}{ 7} = \frac{ 4 \times \frac{5}{2} + 11 } { 7} = \frac{ 21}{7} = 3. \] Now, substitute \( z = \frac{5}{2}\) and \(y = 3 \) into \( x = 6 - 3y + z \) to obtain \[ x = 6 - 3 \times 3 + \frac{5}{2} = - \frac{1}{2}. \]
- Step 5: Hence, the solution is \[ (x,y,z) = \left( - \frac{1}{2}, 3, \frac{5}{2} \right). \ _\square \]
Elimination method
We are given the same system of linear equations as above: \[ \begin{align} x + 3y - z &= 6\\ 2x - y + 2z &= 1\\ 3x + 2y - z &= 2. \end{align}\]
Step 1: Let's eliminate \(x\) from the equations.
Step 2. Twice of the first equation minus the second is \[ \begin{align}
2(x + 3y - z &= 6)\\ -(2x - y + 2z &= 1)\\ \hline 7y - 4z & = 11. \qquad (4) \\\end{align} \] Thrice of the first equation minus the third is \[ \begin{align} 3(x + 3y - z &= 6)\\ -(3x + 2y - z &= 2)\\ \hline 7y - 2z & = 16. \qquad (5) \\ \end{align} \]We need to repeat till we just have one variable.
Step 1. Let's eliminate \( y \) from the equations.
Step 2. The fourth equation minus the fifth is \[ \begin{align} 7y - 4z & = 11 \\ - ( 7y - 2z & = 16 ) \\ \hline -2z & = - 5. \qquad (6) \\ \end{align} \]
Step 3. We are now down to one variable. Solving it gives us \( z = \frac{-5}{-2} = \frac{5}{2} \).
Step 4. Substitute \( z = \frac{5}{2} \) into the fourth equation \( 7y - 4z = 11 \) to obtain \[ y = \frac{ 4z+ 11}{ 7} = \frac{ 4 \times \frac{5}{2} + 11 } { 7} = \frac{ 21}{7} = 3. \] Now, substitute \( z = \frac{5}{2} , y = 3 \) into the first equation \( x = 6 - 3y + z \) to obtain \[ x = 6 - 3 \times 3 + \frac{5}{2} = - \frac{1}{2}. \]
Step 5. Hence, the solution is \[ (x,y,z) = \left( - \frac{1}{2}, 3, \frac{5}{2} \right).\ _\square \]
Solve the following system of equations: \[\]
\[ \begin{align} x + 2y - 3z &= -3\\ 2x - 5y + 4z &= 13\\ 5x + 4y - z&= 5. \\ \end{align} \]
Let us start with the last equation. Solving for \(z\), we obtain
\[z = 5x + 4y - 5.\]
Substituting this into the second equation gives
\[ \begin{align} 2x - 5y + 4(5x + 4y - 5 ) &= 13 \\ 22x + 11y - 33 &= 0 \\ 2x + y - 3 &= 0. \end{align} \]
Substituting this into the first equation gives
\[ \begin{align} x + 2y - 3(5x + 4y - 5) &= -3 \\ x + 2y - 15x -12y + 15 + 3 &= 0 \\ -14x - 10y + 18 &= 0\\ -7x - 5y + 9 &= 0. \end{align} \]
Thus, we now have reduced our system into a pair of equations with two variables:
\[ \begin{align} 2x + y - 3 &= 0\\ -7x - 5y + 9 &= 0. \end{align} \]
Solving for \(y\) in the first equation, we get
\[ y = 3 - 2x. \]
Substituting this into the second equation gives
\[ \begin{align} -7x - 5(3-2x)+9&=0 \\ -7x-15 + 10x + 9 &= 0\\ 3x - 6 &= 0 \\ x &= 2. \end{align} \]
Hence,
\[y = 3 - 2\cdot2 = -1, z = 5\cdot2 + 4\cdot(-1) -5 = 1. \]
Thus, the values of \(x, y,\) and \(z\) which satisfy the given system of equations are \((2,-1,1). \ _\square \)
System of Linear Equations Word Problems - Basic
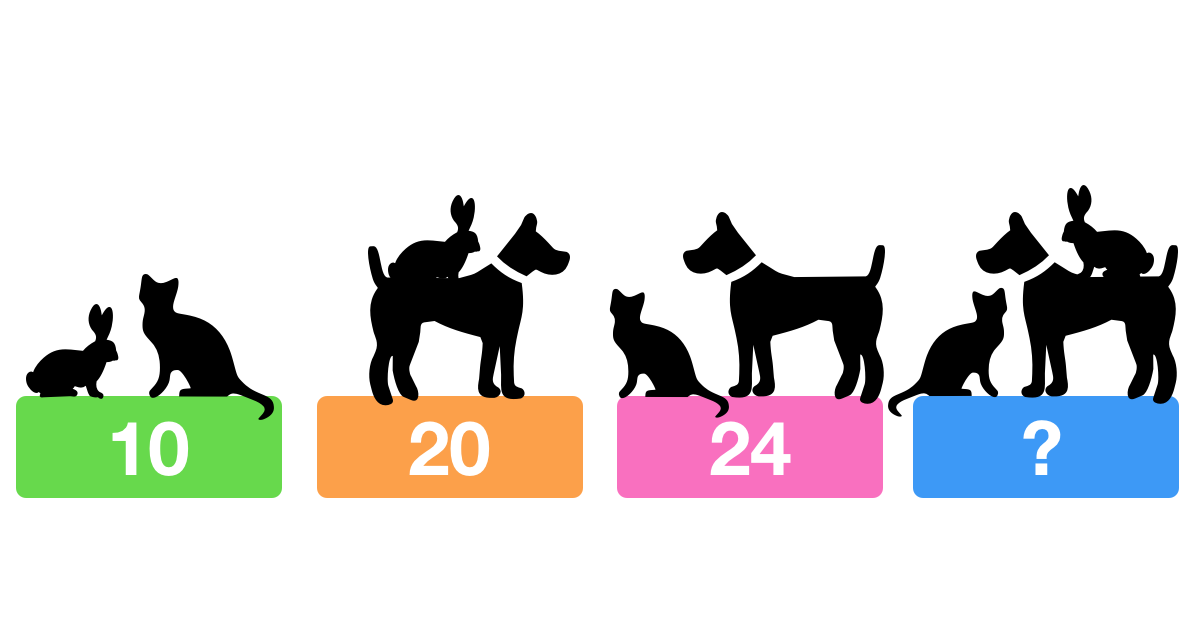
What weight will the fourth scale display?

A bottle that fully contains honey weighs 1500 grams.
The bottle with half of the honey weighs 900 grams.
What is the weight of the empty bottle in grams?
 Today, in a 10-member committee, an old member was replaced by a young member. As such, the average age is the same today as it was 4 years ago.
Today, in a 10-member committee, an old member was replaced by a young member. As such, the average age is the same today as it was 4 years ago.
What is the (positive) difference in ages between the new member and the replaced old member?
System of Linear Equations Word Problems - Intermediate
The brothers Luiz and Lucio bought a land surrounded by a wall of \(340 \text{ meters} \). They built an inner wall to divide the land into two parts. Now, the part of Luiz is surrounded by a wall of \(260 \text{ meters} ,\) and the part of Lucio by a wall of \(240 \text{ meters} \). What is the length of the inner wall?
 Today, there are 4 times as many days to the end of my exams, as there are to the start of my exams.
Today, there are 4 times as many days to the end of my exams, as there are to the start of my exams.
Tomorrow, there are 5 times as many days to the end of my exams, as there are to the start of my exams.
How many days do my exams last for?

There was a thief who went to a wine shop to steal wine. He went in at 10 pm, stole 15 L of wine and added 15 L of water to top up the barrel. He came back at 1 am, stole 15 L of the mixture from the same barrel and added 15 L of water to top it up. He came back at 4 am, stole 15 L of the mixture from the same barrel and added 15 L of water to top it up. In the morning, the ratio of wine to water in the barrel was 343:169. Find the initial amount of wine in the barrel.
System of Linear Equations - Problem Solving
The solutions of the system of equations
\[3x−y=a, \qquad x+y=5\] are the same as those of the system of equations \[2x+y=24, \qquad x−3y=b.\]
What is the value of \(a+b\)?
Since the two systems of equations have the same solutions, we first use substitution to find the solutions to the simultaneous equations
\[x+y=5, \qquad 2x+y=24.\]
Solving the first equation for \(y\) gives \(y=5-x\) and plugging into the second equation gives \(2x + (5-x) = 24\), or \(x = 19\). Plugging this into the first equation gives \(19+y=5\), or \(y=-14\). Thus, \(x=19\) and \( y=−14. \) Substituting these values into \(3x−y=a\) gives
\[a=3x−y=3×19−(−14)=71.\]
Similarly, substituting into \(x−3y=b\) gives
\[b=x−3y=19−3×(−14)=61.\]
Therefore, \[a+b=71+61=132. \ _\square\]
 If the numbers alongside each column and row are the total of the values of the symbols within that column and row, then which number should replace the question mark?
If the numbers alongside each column and row are the total of the values of the symbols within that column and row, then which number should replace the question mark?
Given that \(x=y=0\) is not the only solution to the following system of linear equations, determine all the possible values of \(k:\) \[\begin{align} x+2y &= kx \\ 2x+y &= ky . \end{align}\]