Maxwell's Equations

Maxwell's equations are a set of four differential equations that form the theoretical basis for describing classical electromagnetism:

• Gauss's law: Electric charges produce an electric field. The electric flux across a closed surface is proportional to the charge enclosed.
• Gauss's law for magnetism: There are no magnetic monopoles. The magnetic flux across a closed surface is zero.
• Faraday's law: Time-varying magnetic fields produce an electric field.
• Ampère's law: Steady currents and time-varying electric fields (the latter due to Maxwell's correction) produce a magnetic field.

First assembled together by James Clerk 'Jimmy' Maxwell in the 1860s, Maxwell's equations specify the electric and magnetic fields and their time evolution for a given configuration.

The Lorentz law, where \( q \) and \( \mathbf{v} \) are respectively the electric charge and velocity of a particle, defines the electric field \( \mathbf{E} \) and magnetic field \( \mathbf{B} \) by specifying the total electromagnetic force \( \mathbf{F} \) as

\[ \mathbf{F} = q\mathbf{E} + q\mathbf{v} \times \mathbf{B}. \]

In essence, one takes the part of the electromagnetic force that arises from interaction with moving charge (\( q\mathbf{v} \)) as the magnetic field and the other part to be the electric field.

Gauss's law: The earliest of the four Maxwell's equations to have been discovered (in the equivalent form of Coulomb's law) was Gauss's law. In its integral form in SI units, it states that the total charge contained within a closed surface is proportional to the total electric flux (sum of the normal component of the field) across the surface:

\[ \int_S \mathbf{E} \cdot d\mathbf{a} = \frac{1}{\epsilon_0} \int \rho \, dV, \]

where the constant of proportionality is \( 1/\epsilon_0, \) the reciprocal of the electric constant. The total charge is expressed as the charge density \( \rho \) integrated over a region.

Gauss's law for magnetism: Although magnetic dipoles can produce an analogous magnetic flux, which carries a similar mathematical form, there exist no equivalent magnetic monopoles, and therefore the total "magnetic charge" over all space must sum to zero. Therefore, Gauss' law for magnetism reads simply

\[ \int_S \mathbf{B} \cdot d\mathbf{a} = 0. \]

Faraday's law: The electric and magnetic fields become intertwined when the fields undergo time evolution. In the 1820s, Faraday discovered that a change in magnetic flux produces an electric field over a closed loop. This relation is now called Faraday's law:

\[ \int_\text{loop} \mathbf{E} \cdot d\mathbf{s} = - \frac{d}{dt} \int_S \mathbf{B} \cdot d\mathbf{a}. \]

With the orientation of the loop defined according to the right-hand rule, the negative sign reflects Lenz's law.

Ampère's law: Finally, Ampère's law suggests that steady current across a surface leads to a magnetic field (expressed in terms of flux). In addition, Maxwell determined that that rapid changes in the electric flux \( (d/dt) \mathbf{E} \cdot d\mathbf{a} \) can also lead to changes in magnetic flux. Altogether, Ampère's law with Maxwell's correction holds that

\[ \int_{\text{loop}} \mathbf{B} \cdot d\mathbf{s} = \mu_0 \int_S \mathbf{J} \cdot d\mathbf{a} + \mu_0 \epsilon_0 \frac{d}{dt} \int_S \mathbf{E} \cdot d\mathbf{a}. \]

In summary,

• Gauss' law: \[ \int_S \mathbf{E} \cdot d\mathbf{a} = \frac{1}{\epsilon_0} \int \rho \, dV \]
• Gauss' law for magnetism: \[ \int_S \mathbf{B} \cdot d\mathbf{a} = 0 \]
• Faraday's law: \[ \int_\text{loop} \mathbf{E} \cdot d\mathbf{s} = - \frac{d}{dt} \int_S \mathbf{B} \cdot d\mathbf{a} \]
• Ampère's law: \[ \int_{\text{loop}} \mathbf{B} \cdot d\mathbf{s} = \mu_0 \int_S \mathbf{J} \cdot d\mathbf{a} + \epsilon_0 \mu_0 \frac{d}{dt} \int_S \mathbf{E} \cdot d\mathbf{a}. \]

In their integral form, Maxwell's equations can be used to make statements about a region of charge or current.

To make local statements and evaluate Maxwell's equations at individual points in space, one can recast Maxwell's equations in their differential form, which use the differential operators div and curl.

Differential form of Gauss's law: The divergence theorem holds that a surface integral over a closed surface can be written as a volume integral over the divergence inside the region. Thus,

\[ \frac{1}{\epsilon_0} \int \int \int \rho \, dV = \int_S \mathbf{E} \cdot d\mathbf{a} = \int \int \int \nabla \cdot \mathbf{E} \, dV. \]

Since the statement is true for all closed surfaces, it must be the case that the integrands are equal and thus

\[ \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0}. \]

(The derivation of the differential form of Gauss's law for magnetism is identical.)

Differential form of Ampère's law: One can use Stokes' theorem to rewrite the line integral \( \int \mathbf{B} \cdot d\mathbf{s} \) in terms of the surface integral of the curl of \( \mathbf{B}: \)

\[ \int_\text{loop} \mathbf{B} \cdot d\mathbf{s} = \int_\text{surface} \nabla \times \mathbf{B} \cdot d\mathbf{a}. \]

Ampère's law says that

\[ \int_{\text{loop}} \mathbf{B} \cdot d\mathbf{s} = \mu_0 \int_S \mathbf{J} \cdot d\mathbf{a} + \mu_0 \epsilon_0 \frac{d}{dt} \int_S \mathbf{E} \cdot d\mathbf{a}. \]

Of course, the surface integral in both equations can be taken over any chosen closed surface, so the integrands must be equal:

\[ \nabla \times \mathbf{B} = \mu_0 \mathbf{J} + \mu_0 \epsilon_0 \frac{\partial \mathbf{E}}{\partial t}. \]

Differential form of Faraday's law: It follows from the integral form of Faraday's law that

\[ \int_\text{loop} \mathbf{E} \cdot d\mathbf{s} = - \frac{d}{dt} \int_S \mathbf{B} \cdot d\mathbf{a}. \]

As was done with Ampère's law, one can invoke Stokes' theorem on the left side to equate the two integrands:

\[ \int_S \nabla \times \mathbf{E} \cdot d\mathbf{a} = - \frac{d}{dt} \int_S \mathbf{B} \cdot d\mathbf{a}. \]

Again, one argues that since the relationship must hold true for any arbitrary surface \( S \), it must be the case that the two integrands are equal and therefore

\[ \nabla \times \mathbf{E} = -\frac{d\mathbf{B}}{dt}. \]

In all, the result is as follows:

• Gauss's law \[ \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0} \]
• Gauss's law for magnetism \[ \nabla \cdot \mathbf{B} = 0 \]
• Faraday's law \[ \nabla \times \mathbf{E} = -\frac{d\mathbf{B}}{dt} \]
• Ampère's law \[ \nabla \times \mathbf{B} = \mu_0 \mathbf{J} + \mu_0 \epsilon_0 \frac{\partial \mathbf{E}}{\partial t}. \]

By assembling all four of Maxwell's equations together and providing the correction to Ampère's law, Maxwell was able to show that electromagnetic fields could propagate as traveling waves. In other words, Maxwell's equations could be combined to form a wave equation. Maxwell's insight stands as one of the greatest theoretical triumphs of physics. A simple sketch of this result is as follows:

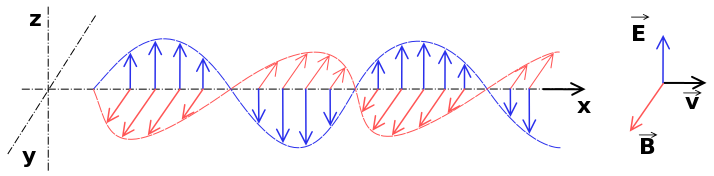
For simplicity, suppose there is some region of space in which the electric field \( E(x) \) is non-zero only along the \( z \)-axis and the magnetic field \( B(x) \) is non-zero only along the \( y \)-axis, such that both are functions of \( x \) only. Then Faraday's law gives

\[ \frac{\partial E}{\partial x} = -\frac{\partial B}{\partial t}. \]

Even though \( \mathbf{J} = 0 \), with the additional term, Ampere's law now gives

\[ \frac{\partial B}{\partial x} = -\frac{1}{c^2} \frac{\partial E}{\partial t}. \]

Taking the partial derivative of the first equation with respect to \( x \) and the second with respect to \( t \) yields

\[\begin{align} \frac{\partial^2 E}{\partial x^2} &= -\frac{\partial^2 B}{\partial x \partial t} \\\\ \frac{\partial^2 B}{\partial t \partial x} &= -\frac{1}{c^2} \frac{\partial^2 E}{\partial t^2}. \end{align} \]

Therefore,

\[ \frac{\partial^2 E}{\partial x^2} = \frac{1}{c^2} \frac{\partial^2 E}{\partial t^2}. \]

This equation has solutions for \( E(x) \) \(\big(\)and corresponding solutions for \( B(x)\big) \) that represent traveling electromagnetic waves. In fact, the equation that has just been derived is in fact in the same form as the classical wave equation in one dimension. In other words, the laws of electricity and magnetism permit for the electric and magnetic fields to travel as waves, but only if Maxwell's correction is added to Ampère's law. Indeed, Maxwell was the first to provide a theoretical explanation of a classical electromagnetic wave and, in doing so, compute the speed of light.

(The general solution consists of linear combinations of sinusoidal components as shown below.)

[1] Griffiths, D.J. Introduction to Electrodynamics. Fourth edition. Pearson, 2014.

[2] Purcell, E.M. Electricity and Magnetism. Third edition. Cambridge University Press, 2013.