Octet Rule

The octet rule reflects the observation that the most stable ions of many elements have eight electrons in their valence shell for gaining the best possible stability.

Chlorine, for example, typically forms an anion with a charge of \(-1\), while sodium typically forms a cation with a charge of \(+1\). These patterns can be used to predict how elements will combine to form polyatomic ions and compounds. These trends can be used to predict the formula unit for compounds. For example, sodium forms the salt \(\ce{NaCl}\) (an ionic compound) when it combines with chloride because its stable ion has a charge of \(+1\), but magnesium will form \(\ce{MgCl_2}\).

The same principle applies when atoms form covalent bonds: the atoms share electrons in such a way that both atoms have a filled outer valence, generally containing an octet of electrons. Negative ions have more electrons than the neutral element, while positive ions have fewer electrons than the neutral element.

The most stable electron configurations for a given element occur when the outermost shell (determined by the principal quantum number) is full.

Returning to the examples listed in the introduction, chlorine has seven electrons in its outer shell, so it is more energetically favorable for chlorine atoms to gain one electron and form a closed shell (as seen in the anion \(\ce{Cl^-}\)) than to shed seven electrons. The halide ion then has the same electron configuration of the noble gas immediately to its right on the periodic table. Similarly, alkali metals have a single valence electron outside of a closed shell, which they readily lose to form a cation with a \(+1\) charge and the electron configuration of the noble gas.

The only elements that have eight valence electrons at ground state are the noble gases (group 18). For example, neon, which has 10 electrons, has the following electron configuration: \[_{10}\text{Ne: } 1s^22s^22p^6 (2, 8).\] Observe that there are \(2+6=8\) electrons in the second shell, which is the outermost shell. The same applies for argon, krypton, and xenon:

\[\begin{align} _{18}\text{Ar:} &\ 1s^2 2s^2 2p^6 3s^2 3p^6 (2, 8, 8)\\ _{36}\text{Kr:} &\ 1s^2 2s^2 2p^6 3s^2 3p^6 4s^2 3d^{10} 4p^6 (2, 8, 18, 8)\\ _{54}\text{Xe:} &\ 1s^2 2s^2 2p^6 3s^2 3p^6 4s^2 3d^{10} 4p^6 5s^2 4d^{10} 5p^6 (2, 8, 18, 18, 8). \end{align}\]

Note: The electron configuration in the brackets is in correspondence to the K, L, M, N system. These three atoms also share the fact that they have \(8\) electrons in their outermost shells.

The stable octet explains why noble gases rarely participate in chemical reactions. They have reached maximum stability without gaining or losing electrons.

There is another rule, called the duplet rule, that states that some elements can be stable with two electrons in their shell.

Hydrogen and helium are special cases that do not follow the octet rule but the duplet rule. They contain an \(s\) orbital but no \(p\) orbitals. They are stable in a duplet state instead of an octet state.

Duplet rule:

Hydrogen and helium have filled their outermost shell and reached a stable configuration when they have two electrons. Having two electrons in the outer valence shell, rather than eight, does not affect the stability of the individual atom, as they have only a 1s orbital, which can hold a maximum of two electrons. These elements do not attain an octet state because the s orbital is too small to accommodate \(8\) electrons and there is a high energy barrier to adding electrons to an empty shell.

The electronic configurations of hydrogen and helium are as follows:

\[\begin{align} _{1}\text{H:} &\ 1s^1 (1)\\ _{2}\text{He:} &\ 1s^2 (2). \end{align}\]

Most free monatomic ions existing in nature obey the octet rule. Monatomic ions gain or lose electrons to have the electron configuration of the noble gas closest in atomic number.

Sodium, or \(_{11}\ce{Na},\) has the following electron configuration:

\[1s^2 2s^2 2p^6 3s^1(2, 8, 1).\]

As discussed above, it has one electron in its outermost shell. Note that the sodium ion now has the electron configuration \(1s^2 2s^2 2p^6(2, 8)\). This is the electron configuration of \(_{10}\text{Ne},\) which is the closest noble gas in atomic number.

Oxygen, or \(_8\ce{O},\) has the following electron configuration:

\[1s^2 2s^2 2p^4(2, 6).\]

It has \(2+4=6\) electrons in its outermost shell. In order to have a stable octet, an oxygen atom can either gain two electrons or lose six electrons. The ionic form of oxygen is \(\text{O}^{2-},\) which also has the electron configuration \(1s^2 2s^2 2p^6.\) This is the same electron configuration adopted by the sodium ion, and \(_{10}\text{Ne},\) which is again the closest noble gas in atomic number.

State the most probable ionic form of calcium (atomic number 20).

ANSWER

The electron configuration of \(_{20}\text{Ca}\) is:

\[1s^2 2s^2 2p^6 3s^2 3p^6 4s^2\]

Calcium has two valence electrons. In order to have eight valence electrons, a calcium atom can either gain six electrons, or lose two electrons. Losing two electrons is more favorable, giving an ionic form of \(_{20}\text{Ca}^{2+}\), which has the same electron configuration as \(_{18}\text{Ar}.\)

The octet rule also applies when covalent bonds are formed. Electrons are shared between the atoms in such a way that they each attain the octet (or duplet) state.

A covalent bond is a chemical bond where electron pairs are shared between two atoms. These electron pairs are known as bonded pairs. They create an equilibrium between the attractive and repulsive forces in a molecule.

This Lewis structure illustrates covalent bonds. Both the carbon and the oxygen have fulfilled their outermost shell and have successfully attained the octet state. The electrons in the carbon-oxygen bonds spend some time orbiting around the nucleus of oxygen and some around the nucleus of carbon.

Check whether \(\ce{SO2}\)(sulfur dioxide) obeys the octet rule. Draw the dot structure confirming the result.

ANSWER

The electron configurations for the elements involved are: \[\begin{align} _{16}\text{S:} &\ 1s^2 2s^2 2p^6 3s^2 3p^4(2, 8, 6)\\ _{8}\text{O:} &\ 1s^2 2s^2 2p^4 (2, 2, 4)\\ \end{align}\] Sulfur requires two electrons to attain the octet state. Oxygen also requires two electrons to attain a stable configuration.

Hence, the sulfur atom shares two electrons each with two oxygen atoms and forms a stable covalent compound. The dot structure confirms the above analysis.

Lewis structures following the octet rule provide a simple model of bonding that is accurate for many compounds, but there are exceptions.

Expanded Valence Octets:

sulfur hexafluoride

Elements in period 3 and beyond can have more than eight electrons in their outer valence. The octet rule is founded on the idea that a valence shell has one \(ns\) orbital and three \(np\) orbitals, each of which can hold two electrons. Starting with \(n=3\), the valence shell also contains five \(nd\) orbitals that can accommodate additional electrons.

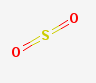sulfur dioxide

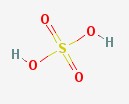sulfuric acid

A common example is sulfur, which forms \( 4\) or \(6\) bonds more often than the characteristic \(2\) that would be predicted based on its location in group 6, with oxygen (oxygen mostly has an oxidation state of \(-2\)).

When sulfur bonds with fluorine, for example, it forms sulfur hexafluoride, with each of the halogens bonded to the central sulfur atom. Every fluorine atom has an octet, giving the sulfur a total of 12 electrons.

Other sulfur-containing compounds behave differently. The sulfur atom has \(4\) bonds (\(2\) sigma and \(2\) pi) in sulfur dioxide, but forms \(6\) bonds (\(4\) sigma and \(2\) pi) in sulfuric acid.

There are many other exceptions. Osmium can form up to 8 bonds!

\[\] Incomplete Octets:

Elements with very light s- and p-block elements (particularly beryllium and boron) can be stable without a complete octet.

In quantum mechanics, electrons have four quantum numbers, n, l, ml, and ms:

• \(n: 1,2,3, ...\)
• \(l: 0,1,2, ... , n-1\)
• \(m_l: -l, -l+1, ... l-1, l\)
• \(m_s: \pm \frac{1}{2}\)

All electrons in the outer shell can't share the same quantum numbers.

So, for \(n=2\), we have the following eight possibilities for \((n,l,m_l,m_s)\):

• \((2,0,0,\frac{1}{2})\)
• \((2,0,0,-\frac{1}{2})\)
• \((2,1,-1,\frac{1}{2})\)
• \((2,1,-1,-\frac{1}{2})\)
• \((2,1,0,\frac{1}{2})\)
• \((2,1,0,-\frac{1}{2})\)
• \((2,1,1,\frac{1}{2})\)
• \((2,1,1,-\frac{1}{2})\)

Thus, we see an example here of the Quantum Mechanical justification of the Octet Rule.

• Lewis Structure
• Bohr's Model
• Coordinate Bonds